Meaning of Wavefunctions

Measured Value

All observable quantities have their operators. $$\hat{A}\phi = a\phi$$ $\phi$ is called the eigenstates or eigenfunction of $\hat{A}$ and $a$ is the eigenvalue of $\hat{A}$
The observed quantity of an observable quantities must be the eigenvalues of $\hat{A}$, hence must be real (if the value is imaginary, we cannot observe it in the real world). The eigenfunction should be othonormal.
e.g. The operator of $p$ is $\frac{\hbar}{i}\frac{d}{dx}$. $$\frac{\hbar}{i}\frac{d}{dx}e^{ikx} = \frac{\hbar}{i}(ik)e^{ikx} = pe^{ikx}$$ Hence, its eigenfunction is $e^{ikx}$ while $p$, the eigenvalue, is the measured value.
e.g. The operator of $x$ is $x$. $$x\delta(x - x_0) = x_0\delta(x - x_0)$$ Hence, its eigenfunction is $\delta(x - x_0)$ while $x_0$, the eigenvalue, is the measured value.

Probability of Measurement

The state of a particle at a scale where quantum effect is significant is given by the wavefunction $\psi$. Information of any observable quantities can be found in the wavefunction.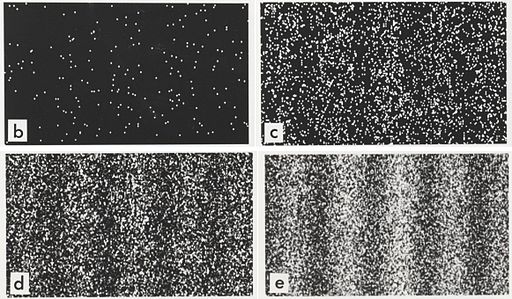

■ Results of a double-slit experiment done by Dr. Tanamura that illustrate the build-up of interference pattern of single electrons

Any wavefunction can be expressed as linear combinations of the eigenfunctions of an observable quantity $$\psi(x) = \sum_{i}c_i\phi_i(x;A)$$ where $|c_A|^2$ is the probability of collapsing to state $\phi_i(x:A)$ and get $A_i$ in $\hat{A}\phi_i(x;A) = A_i\phi_i(x;A)$ upon measuring $A$
Or if $A$ is continuous $$\psi(x) = \int c(A)\phi(x;A)dA$$ where $|c(A)|^2$ is the probability density. e.g. $\psi(x) = \int \psi(x)\delta(x)dx$ The probability density is $$|\psi(x)|^2$$ The probability of finding $x$ between $x_1$ and $x_2$ is $$\int_{x_1}^{x_2} |\psi(x)|^2 dx$$

Collapse of State

After measuring $A$ to be $A_i$ in $\hat{A}\phi_i(x;A) = A_i\phi_i(x;A)$, the state is collapsed to $$\psi = \phi_i(x;A)$$ If $\hat{B}$ commutes with $\hat{A}$, i.e. $$[\hat{A},\hat{B}] = \hat{A}\hat{B} - \hat{B}\hat{A} = 0$$ then the value of $B$ is also determined.
If not, $$\psi = \sum_i c_i\xi_i(x;B)$$ for some non-zero $c_i$
and $B$ is not uniquely determined. If $B$ is then measured to be $B_i$ in $\hat{B}\xi_i(x;B) = B_i\xi_i(x;B)$, the state is collapsed to $\psi = \xi_i(x;B)$. Then, the state is altered from $\psi = \phi_i(x;A)$ to $$\psi = \xi_i(x;B) = \sum_i c_i\phi_i(x;A)$$ for some non-zero $c_i$
$A$ will become not uniquely determined.

Expectation Value

Expectation value is calculated by $\sum_i P_iA_i$ where $P_i$ is the probability of getting $A_i$. $$\begin{align} \psi(x) &= \sum_{i}c_i\phi_i(x;A) \\ \hat{A}\psi(x) &= \sum_{i}c_i\hat{A}\phi_i(x;A) \\ &= \sum_{i}c_iA_i\phi_i(x;A) \\ \psi(x)^*\hat{A}\psi(x) &= \psi(x)^*\sum_{i}c_iA_i\phi_i(x;A) \\ &= (\sum_{i}c_iA_i\phi_i(x;A)^*)(\sum_{i}c_iA_i\phi_i(x;A)) \\ &= \sum_{i}|c_i|^2A_i \\ \end{align}$$ as $\phi_i$ are othonormal.
If $A$ is continuous, then the expectation value is defined as $$\int \psi^* \hat{A} \psi dA$$